Reverberation Time

The reverberation time of a room characterizes how long acoustic energy remains in a room. It is usually defined as the time for the acoustic intensity (or energy density) to decrease by a factor of one million (60 dB). Since a reasonably loud clap is about 100 dB (SPL) and a whisper is about 40 dB, you can easily estimate the reverberation time for a room by clapping and listening to how long you can still hear some remaining sound from the clap. This assumes that the room is not particularly unusual in its dimensions and that it is reasonably quiet.

A simple formula to estimate the reverberation time for a room can be derived by considering a simple model for the room. Start with a room which has a length L, width W, and height H made with walls which are perfectly reflecting. On one wall, there is a window with total area A. If the sound goes out the window, it is lost, otherwise it just bounces off the walls. The window is a model for an effective absorbing area A.

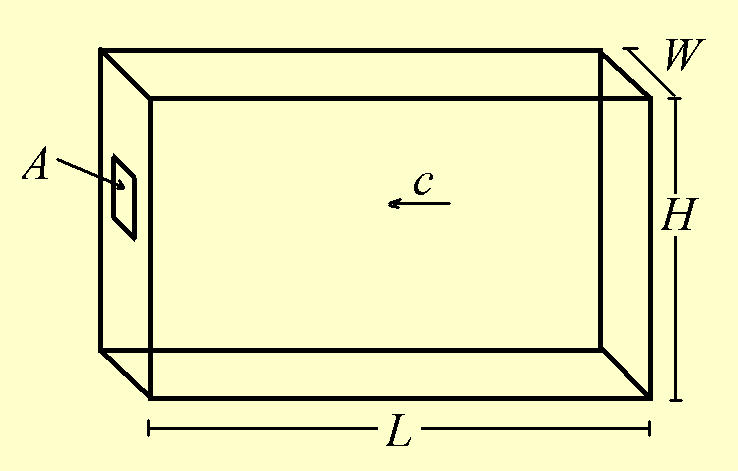
Consider the sound traveling horizontally along the length L with speed of sound, c. The total time for the sound to travel from the wall with the window, across the room, and then return is 2L/c. Each time it does this, the fraction of the sound wave which hits the window is lost. That is, a fraction A/WH is lost. WH is, of course, the area of the wall with the window.

We want to find the time when one millionth of the original energy is left. That is, find the value of nT when (1-A/WH)ⁿ = 1/1,000,000. Taking the log (base ten) of both sides, we get
n log(1-A/WH) = -6
If A/WH is much smaller than one, that is A/WH << 1, then we can use the approximation
log(1-A/WH) = -A/WH .
The room absorption is usually small enough for this to be a good approximation for any case where reverberation time is an issue. Solving for n,
n = 6 WH/A
Hence, nT is then
nT = 12LWH/cA = 12/c times the volume of the room, V = LWH, divided by the (effective) absorbing area, A. You would get this same result if the window was on any of the other walls.

Now, you can expect 1/3rd of the energy to be traveling in each of the three dimensions, so you can expect the loss to be about 1/3rd of this result -- that is, for sound traveling vertically there is no loss from the ceiling and floor, and for sound traveling in the other horizontal direction, from the other two walls. Hence, you expect that the sound energy will remain three times longer and so reverberation time will be
T_R = 36 V/cA.

This approximate formula is very close to the result found experimentally by W. C. Sabine and later derived in more detail by W. S. Franklin (see refs below). The measurements and more careful derivation yields about 55 V/cA. Given the crudity of the model used here, being within a factor of two is quite reasonable. The major difference between the derivation here and the more careful derivation is due to a more accurate treatment for the direction of sound propagation, rather than simply dividing it into three separate dimensions.

The effective absorbing area for a room can be computed from the actual area and the absorption coefficient for that area. Absorption coefficients depend on the frequency of the sound and tables are available for typical materials. Some objects, like chairs, are often characterized by simply using an equivalent aborbing area based on measurement rather than trying to compute it in detail. The equivalent areas are often reported in units of sabins. One sabin corresponds to the same amount of absoption as a one square foot perfectly absorbing "window."

Work continues to refine Sabine's formula to this day. In particular there are some troubling limiting cases. See, for example, the article by K. S. Sum (see refs below).

W. S. Franklin, "Derivation of Equation of Decaying Sound in a Room and Definition of Open Window Equivalent of Absorbing Power," Physical Review (Series I) 16, 372-374 (1903).

K. S. Sum, "Some comments on Sabine absorption coefficient," J. Acoust. Soc. Am. 117, 486-489 (2005)

Questions/Comments to: suits@mtu.edu

There are no pop-ups or ads of any kind on these pages. If you are seeing them, they are being added by a third party without the consent of the author.