Clarinet
A clarinet is an example of a cylindrical bore instrument
closed at one end. Hence, the normal resonant modes must
have a pressure maximum at the closed end (the mouthpiece)
and a pressure minimum near the first open key (or the
bell). These conditions result in the presence of only
odd harmonics in the sound. This contrasts to the saxophone
or oboe, which have a conical bore and hence include the
even harmonics.
A snapshot of the sound of a clarinet (playing Bb) is shown below:
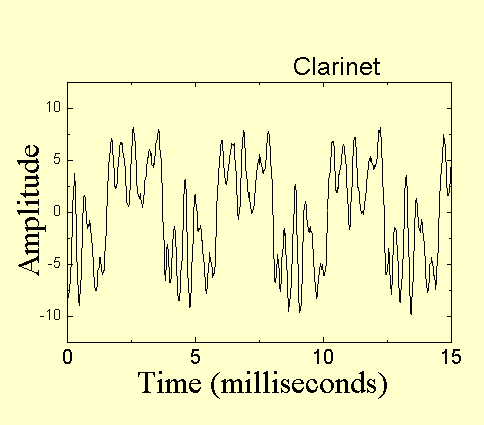
and the absence of the even harmonics in the spectrum is clearly evident.
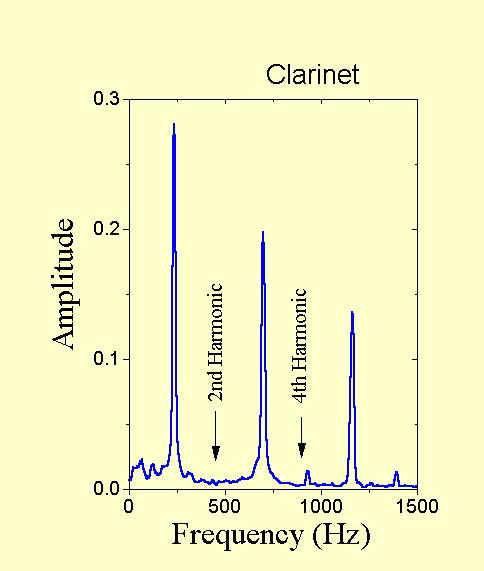
The absence is even harmonics is (part of) what is responsible
for the "warm" or "dark" sound of a clarinet compared to
the "bright" sound of a saxophone.
Click here for
more information on the difference
between cylindrical
and conical bores, or see
"The conical bore in musical acoustics," by R. D. Ayers, L. J. Eliason,
and D. Mahgerefteh, American Journal of Physics, Vol 53, No. 6,
pgs 528-537, (1985).
Here is a WAV file with a recorded clarinet sound:
Clarinet.
You can create your own simulated clarinet sound s(t) as follows:
with w1 = frequency of fundamental (in Hz) times 2 pi, the simulated
clarinet waveform as a function of time, t (in seconds) is:
s(t) = sin(w1t) +
0.75*sin(3*w1t) +
0.5*sin(5*w1t) +
0.14*sin(7*w1t) +
0.5*sin(9*w1t) +
0.12*sin(11*w1t) +
0.17*sin(13*w1t)
and then multiply s(t) by a constant to change the amplitude. The
waveform for the simulated clarinet will look a little different than
that of the recorded clarinet since no phase shifts are included (e.g. only
sine functions are used, and no cosine functions), but it sounds
remarkably similar. Note that the cosine functions are the same
as sine functions shifted in time and for sustained tones, these
"phase shifts" do not change the
sound.
Check it out:
Simulated Clarinet WAV file (fundamental = 235.5 Hz).
There are no pop-ups or ads of any kind on these pages.
If you are seeing them, they are being added by a third
party without the consent of the author.
Questions/Comments to:
suits@mtu.edu
To Physics of Music Notes
To MTU Physics Dept Home Page
Copyright Info